When we have four equations, we have to eliminate variables so we form 2x2 systems, that is, two equations with two variables.
First, multiply the first equation by -1, to then combine it with the second equation.
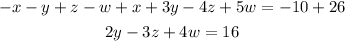
Let's repeat the process with equations 3 and 4.

Repeat the process one more time because we need three equations with the variables y, z, and w. This time let's do it with equations 1 and 3.
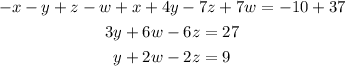
Now, combine the first two equations we've got.
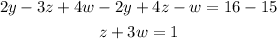
We need another equation with the variables z and w only. To find it, multiply the third equation by 2 and combine it with equation number 2 above.

The value of w is 1.
Let's use the w-value in the equation z+3w=1 to find z.
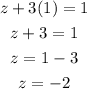
The value of z is -2.
Now we can find the value of y using the equation y+2w-2z=9.
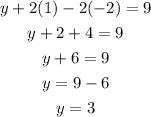
The value of y is 3.
At last, we can find x using any equation because we already know three variables.
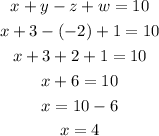
The value of x is 4.
Therefore, the solutions to the system are x = 4, y = 3, z = -2, and w = 1.