Question

Find the slope of the tangent line to the curve 3x ^ 2 + 3xy - 3y ^ 3 = - 51 at the point (2, 3) .

Answer 1

- To find the equation of the tangent, we should:

1. Find the derivative of the equation with respect to x. This give us the expression for all the possible slopes of the curve.

2. Substitute the values of (x, y) = (2, 3) into the expression in step 1. This gives the particular slope of the tangent line.

3. After this, you use the formula below to find the equation of the tangent line:

`\begin{gathered} m(x_1,y_1)=(dy)/(dx)(x_1,y_1)=(y-y_1)/(x-x_1) \\ where, \\ x_1,y_1\text{ is the coordinate that lies both on the line and on the curve. \lparen i.e. the point where the} \\ \text{ tangent touches the curve\rparen} \\ \text{ For our question, }x_1=2,y_1=3 \\ \end{gathered}`

- Thus, we can solve the question as follows:

STEP 1:

`\begin{gathered} 3x^2+3xy-3y^3=-51 \\ \text{ Differentiate with respect to }x \\ \\ (d)/(dx)(3x^2+3xy-3y^3)=(d)/(dx)(-51) \\ \\ (d)/(dx)(3x^2)+(d)/(dx)(3xy)-(d)/(dx)(3y^3)=0 \\ \\ 6x+3x(d)/(dx)(y)+3y(d)/(dx)(x)-3(d)/(dx)(y^3)=0 \\ \\ \text{ Apply the chain rule for for the ''y'' expressions} \\ \\ 6x+3x(dy)/(dx)+3y-3(d)/(dy)(y^3).(dy)/(dx)=0 \\ \\ 6x+3x(dy)/(dx)+3y-9y^2(dy)/(dx)=0 \\ \\ \text{ Factorize out }(dy)/(dx) \\ \\ (dy)/(dx)(3x-9y^2)+6x+3y=0 \\ \\ \text{ Subtract }6x\text{ and }3y\text{ from both sides} \\ \\ (dy)/(dx)(3x-9y^2)=-(6x+3y) \\ \\ \text{ Divide both sides by }(3x-9y^2) \\ \\ \therefore(dy)/(dx)=(-(6x+3y))/((3x-9y^2))=(-3(2x+y))/(3(x-3y^2)) \\ \\ (dy)/(dx)=(2x+y)/(3y^2-x)\text{ \lparen The negative sign was applied to the denominator\rparen} \end{gathered}`

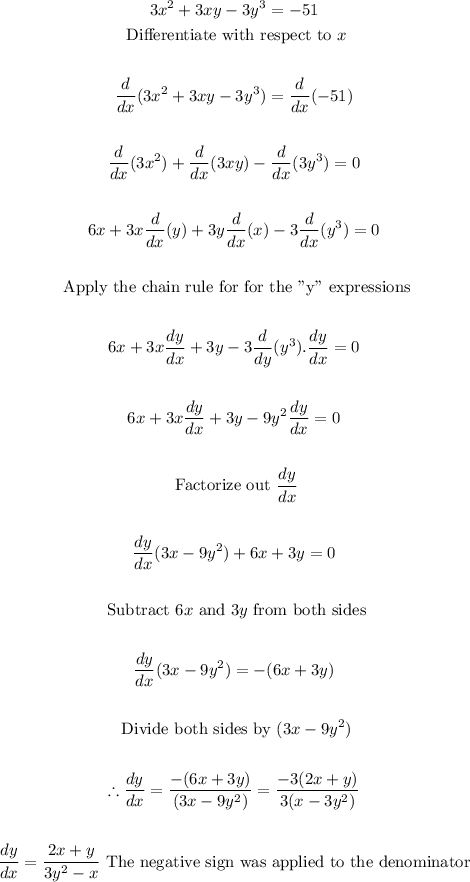
- Now that we have our equation for the slope, let us find the particula slope

STEP 2:

`\begin{gathered} (dy)/(dx)=m(x,y)=(2x+y)/(3y^2-x) \\ \\ put\text{ }(x,y)=(2,3) \\ \\ m=(2(2)+3)/(3(3)^2-2) \\ \\ m=(4+3)/(27-2) \\ \\ m=(7)/(25) \\ \end{gathered}`

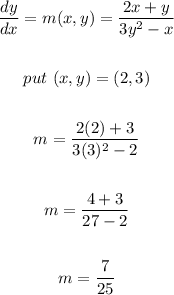
- Now that we have the slope of the tangent line, we can thus find the equation of the tangent line.

Final Answer

The slope of the tangent line is

`(7)/(25)`