Question

What was the initial mass (in mg) of the sample? What is the mass (in mg) 6 weeks after the start?

Answer 1

The formula for the exponential decay is as follows.

`N(t)=N_0\mleft((1)/(2)\mright)^{(t)/(t_((0.5_)))}`

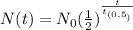
where N(t) is the final value, N₀ is the initial value, t is the time that passed, and t₀.₅ is the half-life.

In given, we have the following.

`\begin{gathered} t=24 \\ t_(0.5)=4 \\ N(t)=3 \\ N_0=\text{?} \end{gathered}`

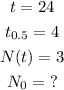
Substituting to the equation, we can solve for N₀.

`\begin{gathered} N(t)=N_0\mleft((1)/(2)\mright)^{(t)/(t_((0.5_)))} \\ 3=N_0\mleft((1)/(2)\mright)^{(24)/(4)} \end{gathered}`

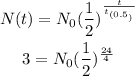
Thus, we have the following.

`\begin{gathered} 3=N_0\mleft((1)/(2)\mright)^6 \\ 3=N_0\mleft((1)/(64)\mright) \\ 192=N_0 \\ N_0=192 \end{gathered}`

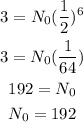
Therefore, the initial mass must be 192 mg.

To solve for the mass in 6 weeks, convert the weeks into days. Note that in 1 week, there are 7 days.

`6\text{ weeks}\cdot\frac{7\text{ days}}{1\text{ week}}=42\text{ days}`

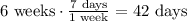
Substitute the given values and the 42 days as t into the equation and then solve for N(t).

`\begin{gathered} N(t)=N_0\mleft((1)/(2)\mright)^{(t)/(t_((0.5)))} \\ N(t)=192\mleft((1)/(2)\mright)^{(42)/(4)} \\ =192\mleft((1)/(2)\mright)^(10.5) \\ \approx192(0.000690533966) \\ \approx0.1325825215 \\ \approx0.1326 \end{gathered}`

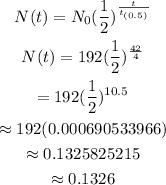
Therefore, in 6 weeks, the mass must be approximately 0.1326 mg.