Question

Find the image C (-1,3) under the transformations T (-2,3) ° r x-axis

Answer 1

First, we have that the equation to calculate the reflection over the x-axis is:

`r_x(x,y)=(x,-y)`

And the forumal for the reflection of point 'a' across the point 'p' is:

`T_p(a)=(2p_1-a_1,2p_2-a_2)`

then, for the point C(-1,3), we have the following:

`\begin{gathered} (T_((-2,3))\circ r_x)(C)=(T_((-2,3))\circ r_x)(-1,3)_{}_{} \\ =T_((-2,3))(r_x(-1,3))=T_((-2,3))(-1,-3)=(2(-2)-(-1),2(3)-(-3)) \\ =(-4+1,6+3)=(-3,9) \end{gathered}`

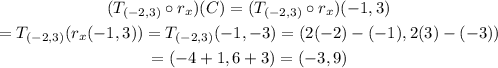
therefore, the image of C(-1,3) under the transformations is (-3,9)