Given,
The mass of the book, m=1.594 kg
The force, F=2.84 N
The angle, θ=20.2°
A)
The book is moving in the horizontal direction with a constant velocity. Thus there is no net force acting on the book in vertical or horizontal directions.
The net force acting on the book in the horizontal direction is given by,

Where f is the frictional force and N is the normal force acting on the book.
The normal force is given by,
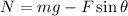
On substituting the known values,
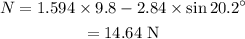
Substituting all the known values in equation (i),
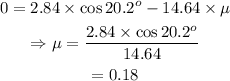
Therefore the coefficient of friction between the book and the table is 0.18
B)
As it can be seen from the diagram, pulling the book with force F at an angle θ will have the same effect as pushing the book with a force F and angle θ. Thus the horizontal and the vertical components of the force F will be the same as it was in (A). Therefore the net force on the book in the vertical or horizontal direction will be zero even in this case.
As the net force on the book in the vertical direction is zero, from Newton's second law,
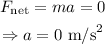
Where a is the acceleration of the book.
Thus the acceleration of the book is 0 m/s²