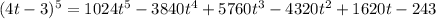Question

(4t-3) to the fifth poweruse the the binomial theorem

Answer 1

The binomial theorem says that

`(x+y)^n=\sum ^n_(k\mathop=0)(n!)/(k!(n-k)!)x^(n-k)y^k`

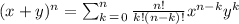
Looking at our problem we can see that

• x = 4t

,

• y = -3

,

• n = 5

Using it in the binomial theorem

`(4t-3)^5=\sum ^5_{k\mathop{=}0}(5!)/(k!(5-k)!)(4t)^(5-k)(-3)^k`

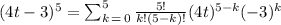
And now we do the calculus for each term, then, I'll do it separated, just to be organized since binomial theorem calculus can be long, I'll start with k = 0 and go on.

k = 0

`\begin{gathered} (5!)/(0!(5-0)!)(4t)^(5-0)(-3)^0=4^5t^5 \\ \\ 4^5t^5=1024t^5 \end{gathered}`

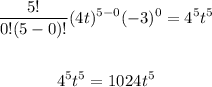
k = 1

`\begin{gathered} (5!)/(1!(5-1)!)(4t)^(5-1)(-3)^1=(5!)/(4!)4^4t^4(-3) \\ \\ (5!)/(4!)4^4t^4(-3)=(-3)\cdot5\cdot256\cdot t^4 \\ \\ (-3)\cdot5\cdot256\cdot t^4=-3840t^4 \end{gathered}`

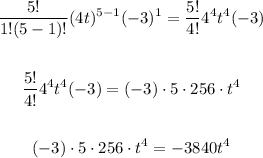
k = 2

`\begin{gathered} (5!)/(2!(5-2)!)(4t)^(5-2)(-3)^2=(5!)/(2!\cdot3!)(4t)^3(-3)^2 \\ \\ (5!)/(2!\cdot3!)(4t)^3(-3)^2=10\cdot64\cdot t^3\cdot9 \\ \\ 10\cdot64\cdot t^3\cdot9=5760t^3 \end{gathered}`

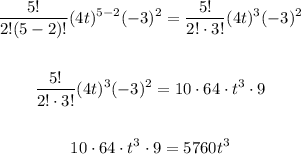
k = 3

`(5!)/(3!(5-3)!)(4t)^(5-3)(-3)^3=-4320t^2`

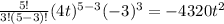
k = 4

`(5!)/(4!(5-4)!)(4t)^(5-4)(-3)^4=1620t^{}`

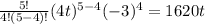
And the last one, k = 5

`(5!)/(5!(5-5)!)(4t)^(5-5)(-3)^5=-243`

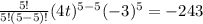
Now we did all terms, the binomial will be the sum of all of them

`(4t-3)^5=1024t^5-3840t^4+5760t^3-4320t^2+1620t-243`