Question

Answer to part 1: 10% is the percent increase and 79,000 is the average salary. Using the current Year's mean annual salary from part 1 calculate the percent of your salary you will pay if your student loan payments are $295.00 per month for 120 months.

Answer 1

We have to calculate the proportion, expressed in percentage, of the salary that is paid in student loan.

The salary is $79,000 and increase 10% annually.

The student loan payments are $295 per month for 120 months.

This is equivalent to 295*12 = $3540 per year.

The period is equivalent to 10 years.

We then can calculate the proportion as the quotient between the total loan payments and the total salary in the 10 year period.

We can calculate the total salary in 10 years as:

`\begin{gathered} S_1=79000 \\ S_2=79000\cdot1.1 \\ S_2=79000\cdot1.1^2 \\ \Rightarrow S=\sum ^(10)_(k=1)S_i \\ S=79000\cdot\sum ^(10)_(k=1)1.1^k \\ S=79000\cdot((1-1.1^(10))/(1-1.1)) \\ S=79000\cdot(1-1.1^(10))/(-0.1) \\ S=79000\cdot(1-2.5937424601)/(-0.1) \\ S=79000\cdot(-1.5937424601)/(-0.1) \\ S=79000\cdot15.937424601 \\ S\approx1259056.54 \end{gathered}`

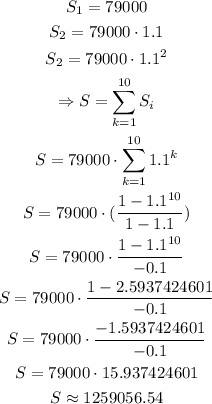
The salary was calculated as the sum of a geometric series wirh ratio r = 1.1, which represents the 10% increase each year.

The total salary in the 10-year period is S = $ 1,259,056.54.

The total payments in students loan can be calculated as:

`P=259\cdot120=31080`

Then we can calculate the proportion as:

`p=(P)/(S)=(31080)/(1259056.54)\approx0.024685=2.47\%`

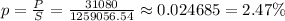
Answer: the percentage paid in students loan is approximately 2.47% of the salary.