Question

33. Suppose that the scores on a statewide standardized test are normally distributed with a mean of 75 and a standard deviation of 4. Estimate the percentage of scores that were (a) between 63 and 87. % (b) above 83. % (c) below 71. % (d) between 67 and 79. %

Answer 1

The mean and standard deviation of the scores are given below:

• Mean = 75

,

• Standard deviation = 4

We make use of the z-score formula below:

`z-\text{score}=(X-\mu)/(\sigma)\text{ where}\begin{cases}\mu=\text{Mean} \\ \sigma=\text{Standard Deviation}\end{cases}`

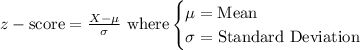
Part A (between 63 and 87)

First, we determine the z-scores.

Therefore, the percentage of scores that were above 83 is 2.28%.

Part C (below 71)

`\begin{gathered} z-\text{score}=(71-75)/(4)=-(4)/(4)=-1 \\ \text{From the z-score table: }P(x<-1)=0.15866 \end{gathered}`

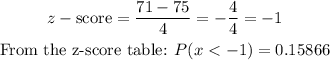
Therefore, the percentage of scores that were below 71 is 15.87%.

Part D (between 67 and 79)

[tex]\begin{gathered} z-score=\frac{67-75}{4}=\frac{-8}{4}=-2 \\ z-score=\frac{79-75}{4}=\frac{4}{4}=1 \\ \text{From the z-score table: }P(-2Therefore, the percentage of scores that were between 67 and 79 is 81.86%.