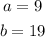Step 1
Given;
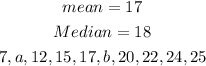
Step 2
The median is given as;
The median is the middle number in a sorted, ascending or descending, list of numbers and can be more descriptive of that data set than the average. The median is sometimes used as opposed to the mean when there are outliers in the sequence that might skew the average of the values.
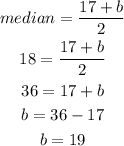
Step 3
The mean is given as;
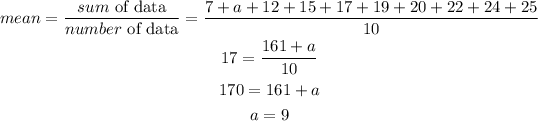
Answer;