Question

Standing on one bank of a river, an explorer measures the angle to the top of a tree on the opposite bank to be 27 degrees. He backs up 50 feet andmeasures the angle to the top of the tree to now be 22 degrees. How wide is the river? Round to the nearest tenth of a foot18.7 feet191.5 feet20.2 feet187.3 feet

Answer 1

191.5 feet

Explanation:

According to the statement, we can plan the following graph that represents the situation:

We can see two right triangles in the figure graph ABC and DBC. Where the width of the river would be x.

We can calculate the value of x with the help of the tangent trigonometric ratio, just like that.

`\begin{gathered} \tan \theta=\frac{\text{ opposite}}{\text{ adjacent}} \\ \text{For ABC} \\ \tan 22=(CB)/(AB) \\ \tan 22=(CB)/(50+x)\rightarrow CB=\tan 22\cdot(50+x)\text{ (1)} \\ \text{For DBC} \\ \tan 27=(CB)/(DB) \\ \tan 27=(CB)/(x)\rightarrow CB=\tan 27\cdot x\text{ (2)} \end{gathered}`

We match both equations so that you can calculate the value of x:

`\begin{gathered} \tan 22\cdot(50+x)=\tan 27\cdot x \\ 50\tan 22+x\tan 22=x\tan 27 \\ x\tan 27-x\tan 22=50\tan 22 \\ x\cdot(\tan 27-\tan 22)=50\tan 22 \\ x=(50\tan 22)/((\tan 27-\tan 22)) \\ x=191.5\text{ ft} \end{gathered}`

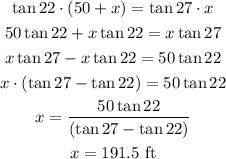
Therefore, the wide of the river is 191.5 feet.