Question

Alex walked on an asphalt pathway for 15 miles. He walked the 15 miles back to his car on a gravel road through the forest. On the asphalt he walked 5 miles per hour faster than on the gravel. The walk on the gravel took 4 hours longer than the walk on the asphalt. How fast did he walk on the gravel?

Answer 1

Given:

Alex walked 15 miles on asphalt pathways.

In asphalt 5 miles per hour more

Time takes more than 4 hours.

Sol:

How fast did he walk on gravel:

Sol:

Speed in gravel = x

then speed in asphalt pathway = x+5

Time taken in asphalt = t

So time taken in gravel = t+4

So speed in Asphalt pathway:

`\text{ Speed=}\frac{\text{ Distance}}{Time}`
`\begin{gathered} x+5=(15)/(t) \\ \\ t=(15)/(x+5) \end{gathered}`

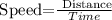
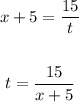
Speed in gravel is:

`\begin{gathered} x=(15)/(t+4) \\ \\ t+4=(15)/(x) \\ \\ t=(15)/(x)-4 \end{gathered}`

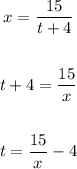
Solve for "x" is:

`\begin{gathered} (15)/(x+5)=(15)/(x)-4 \\ \\ 15x=(x+5)(15-4x) \\ \\ 15x=15x-4x^2-20x+75 \\ \\ 4x^2+20x-75=0 \\ \\ 4x^2+30x-10x-75=0 \\ \\ 2x(2x+15)-5(2x+15)=0 \\ \\ (2x+15)(2x-5)=0 \\ \\ x=-(15)/(2);x=(5)/(2) \end{gathered}`

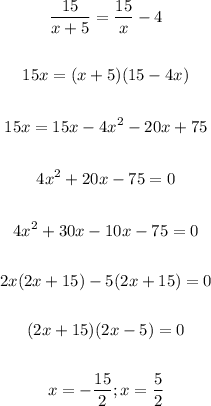
Speed is always positive so speed in gravel is 5/2 or 2.5 miles per hour.