Question

The total revenue for Jane’s Vacation rental is given as the function R(x) = 100x-0.2x squared where x is the number of rooMs filled. What number of rooms filled produced the maximum revenue

Answer 1

Given that

The function of revenue is R(x) = 100x - 0.2x^2

Explanation -

It is given that we have to find the number of rooms filled that gives maximum revenue.

And x is the number of rooms filled and R is the total revenue.

If the [Total revenue] value of the function is maximum it means its derivative is zero so we will find the derivative first.

Derivative of given function

`\begin{gathered} (dR(x))/(dx)=(d)/(dx)(100x-0.2x^2) \\ R^(\prime)(x)=100-0.2**2x=100-0.4x \end{gathered}`

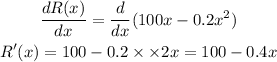
Since derivative of R(x) is zero then,

100 - 0.4x = 0

0.4x = 100

x = 100/0.4 = 1000/4 = 250

So the number of rooms filled to give maximum revenue is 250.

The final answer is 250.