Question

A rubber ball is attached to a 1.44m string and spin in a horizontal circle. The tension in the string is 2.91 N. It takes 0.644s for the ball yo complete one revolution. What is the mass in kg of the ball?

Answer 1

We are given that a mass is attached to a string and is spun is in a horizontal circle, this means that the free body diagram of the problem is the following:

We will add the forces in the vertical direction:

`T_y-mg=0`

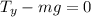
The forces add up to zero since there is no acceleration in the vertical direction. Therefore, we can add "mg" to both sides:

`T_y=mg`

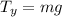
Now, The vertical component of the tension can be put in terms of the total tension using the following right triangle:

Therefore, we use the trigonometric function sine:

`\cos x=(T_y)/(T)`

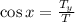
Multiplying both sides by "T"

`T\cos x=T_y`

Substituting in the sum of vertical forces:

`T\cos x=mg`

Now, Since the horizontal component of the tension is equivalent to the centripetal force, we have that:

`T_x=ma_r`

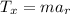
Where:

`\begin{gathered} r=\text{ radius of the circle} \\ a_r=\text{ radial acceleration} \end{gathered}`

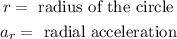
We can use the trigonometric function cosine to determine the horizontal component of the tension:

`\sin x=(T_x)/(T)`

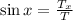
Multiplying both sides by "T":

`T\sin x=T_x`

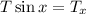
Substituting we get:

`T\sin x=ma_r`

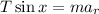
Now, we divide both equations:

`(T\sin x)/(T\cos x)=(ma_r)/(mg)`

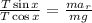
Simplifying we get;

`\tan x=(a_r)/(g)`

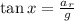
Now, we multiply both sides by "g":

`g\tan x=a_r`

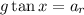
Now, in any circular motion, the period "P" is given by:

`P=(2\pi)/(\omega)`

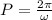
Where:

`\begin{gathered} r=\text{ radius} \\ v=\text{ tangential velocity} \end{gathered}`

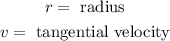
Also, the acceleration is given by:

`a_r=(4\pi^2r)/(P^2)`

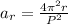
Substituting the expression for the acceleration we determined before we get:

`g\tan x=(4\pi^2r)/(P^2)`

Substituting the expression for the period:

`g\tan x=(4\pi^2r)/(((2\pi)/(\omega))^2)`

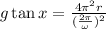
Solving the square:

`g\tan x=(4\pi^2r)/((4\pi^2)/(\omega^2))`

Solving the fraction:

`g\tan x=(4\pi^2r\omega^2)/(4\pi^2)`

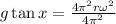
Simplifying:

`g\tan x=r\omega^2`

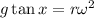
Now, we can put the radius in terms of the length of the spring using the following triangle:

using the function sine we get:

`L\sin x=r`

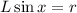
Substituting in the previous equation we get:

`g\tan x=L\sin x\omega^2`

Now, we decompose the tangent:

`(g\sin x)/(\cos x)=L\sin x\omega^2`

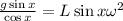
Simplifying:

`(g)/(\cos x)=L\omega^2`

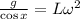
Now, we invert both sides:

`(\cos x)/(g)=(1)/(L\omega^2)`

Multiplying both sides by "g" we get:

`\cos x=(g)/(L\omega^2)`

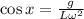
The angular velocity is the angle divided by time, since it takes 0.644 s to complete one revolution this means that :

`\omega=(2\pi)/(0.644)`

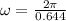
substituting the values:

`\cos x=(9.8)/(1.44((2\pi)/(0.644))^2)`

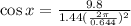
Solving the operations:

`\cos x=0.071`

Now, going back to the sum of forces in the vertical direction:

`T\cos x=mg`

Dividing both sides by "g":

`(T\cos x)/(g)=m`

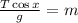
Substituting the values:

`((2.91)(0.071))/(9.8)=m`

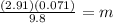
Solving the operations:

`0.021=m`

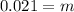
Therefore, the mass of the ball is 0.021 kg.