Question

in the following coordinate plane, find the image of each of the given points in the transformation that is the composition of a reflection in line m followed by a reflection in line n. a. (4,8)b. (0,1)c. (-1,0)d. (0,0)

Answer 1

We want to calculate the image of (4,8). So first we identify that line m is line y=2.

To reflect the point around the line m, we need to find the distance between point (4,8) and line y=2. To do so, we will draw a vertical line that passes through point (4,8) and check where it crosses the line y=2. So the line would look like this.

Now, we want to calculate the distance between this two points. To do so, we will use the formula

`d=\sqrt[]{(a-c)^2+(b-d)^2}`

which would be tghe distance between points (a,b) and (c,d). So using the formula to points (4,8) and (4,2) we get

`d=\sqrt[]{(8-2)^2+(4-4)^2}=\sqrt[]{(8-2)^2}=(8-2)=6`

Now, we want to find a point over the blue line that is at distance 6 from the red line, but is under the red line. We achieve this by taking the point of intercsection (4,2) and subtract 6 on the y coordiante. So the reflection of point (4,8) on line m is simply given by the point

`(4,2-6)=(4,-4)`

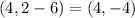
So the reflection of point (4,8) on line m is the point (4,-4).

Now, we want to reflect (4,-4) on line n. First we identify that the vertical line n is the line x=3. Now, we repeat the process as before.

In this case, we draw a horizontal line to identify the point of intersection, it would be

NOw, we calculate the distance. As they are on a horizontal line, we can simply subtract the x coordiantes and then take the absolute value.

This leads to

`d=|4-3|=1`

So know we subtract the distance from the x coordinate of the intersection. We get

`(3-1,-4)=(2,-4)`

So the reflection of point (4,-4) on line n is (2,-4)