Question

1 point Two midsegments of A ABC are DE and DF. B. A and the length of DF ist The length of DB is type your answe answer. ,

Answer 1

Midsegments are lines that conect the midpoints of two sides of a triangle. This means that it divides both sides in two equal segments, for this diagram:

From this we can determine that segments AD and DB are equal.

So segment DB = 10

To calculate segment DF you have to apply the midsegment theorem that states that each midsegment is parallel to the third line (i.e. the side they dont intersect)

For this diagram: DE || AC and DF || BC

Next propperty you have to remember is that each midsegment is a halve of the third side, so for segment DF:

`\begin{gathered} DF=(1)/(2)BC \\ DF=(1)/(2)26 \\ DF=13 \end{gathered}`

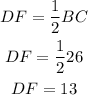
Segment DF=13