Let's put more details in the given graph to better understand the problem:
For the rectangular coordinates, it means that we will be looking for the x and y.
But first, let's determine the polar coordinates of the point.
From the given graph, it appears that the point is at r = 4.
For the angle, let's first determine the measure of each units represented by A.
Since there are 6 units in a 90° angle, let's find A:
6A = 90°
6A/6 = 90°/6
A = 15°
Therefore, Θ will be:
Θ = 4A
= 4(15°)
Θ = 60°
Let's now solve for x and y.
x = r · Cos Θ
x = 4 · Cos (60°)
x = 2
Since it is in the second quadrant, x should be a negative value.
Thus, x = -2
y = r · Sin Θ
y = 4 · Sin (60°)
y = 2√3
Therefore, the rectangular coordinates of P is:
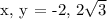
The answer is CHOICE A.