Question

Hi, can you help me answer this question please, thank you!

Answer 1

As your testing H0:p=0.79, then you have a two-tailed test.

The p-value at two-tailed test is given by:

`\begin{gathered} p=P(Z\leq-z)+P(Z\ge z) \\ p=P(Z\leq-z)+(1-P(Z\leq z) \\ p=2P(Z\leq-z) \\ 0.79=2P(Z\leq-z) \\ P(Z\leq-z)=(0.79)/(2) \\ P(Z\leq-z)=0.395 \end{gathered}`

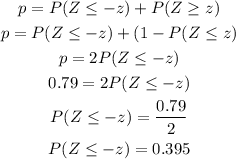
Then, you need to check into the standard normal cumulative table to find at which -z you do have a probability of 0.395. Thus:

As you can see in the picture at z=-0.26 you have a P(Z<=-z)=0.3974

And at z=-0.27 you have a P(Z<=-z)=0.3936.

The average of these values is:

`(0.3974+0.3936)/(2)=0.3955`

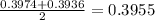
Then, you have a probability of 0.395 at a z of:

`(-0.26+(-0.27))/(2)=-0.265`

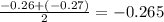
Then -z=-0.27, thus z=0.27