Question

Factorize the following function 36(3x-2)² - 25(2-x)²

Answer 1

The question is given to factorize the expression:

`36\mleft(3x-2\mright)^2\: -\: 25\mleft(2-x\mright)^2​`

Given that:

`\begin{gathered} 36=6^2 \\ 15=5^2 \end{gathered}`

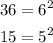
Therefore, the expression becomes:

`\Rightarrow6^2(3x-2)^2\: -\: 5^2(2-x)^2​`

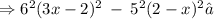
Recall the rule of exponents:

`m^x\cdot n^x=(m\cdot n)^x`

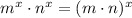
Hence, the expression can be rewritten to be:

`\Rightarrow\lbrack6(3x-2)\rbrack^2\: -\: \lbrack5(2-x)\rbrack^2`

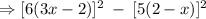
Expand the terms in the brackets:

`\begin{gathered} 6(3x-2)=18x-12 \\ 5(2-x)=10-5x \end{gathered}`

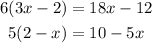
Hence, we have the expression to be:

`\Rightarrow(18x-12)^2-(10-5x)^2`

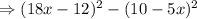
Recall the Difference of Two Squares Formula, defined as:

`x^2-y^2=(x-y)(x+y)`

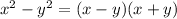
Hence, we have the expression to be:

`(18x-12)^2-(10-5x)^2=\lbrack(18x-12)-(10-5x)\rbrack\cdot\lbrack(18x-12)+(10-5x)\rbrack`

Simplifying, we have:

`\Rightarrow(18x-12-10+5x)\cdot(18x-12+10-5x)=(23x-22)(13x-2)`

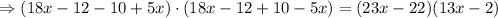
ANSWER:

`36\mleft(3x-2\mright)^(2)-25\mleft(2-x\mright)^(2)​=(23x-22)(13x-2)`