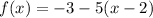Question

Diagram 2 shows the graph of a quadratic function f(x) =3+p (x-r)²Finda) value of p,q and rb) if the graph is reflected about to y-axis, write the equation of the curve

Answer 1

Given the graph of the function:

`f(x)=3+p(x-r)^2`

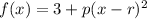
From the graph of the function:

Vertix = (2, q)

The y-intercept = (0, 23)

We will find the values of p, q, and r

From the point of the vertex of the graph: r = 2, q = 3

And from the point of the y-intercept

When x = 0, f(x) = 23 and substitute with r

so,

`23=3+p(0-2)^2`

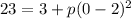
Solve the equation to find p

`\begin{gathered} 23-3=4p \\ 20=4p \\ p=(20)/(4)=5 \end{gathered}`

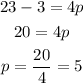
so, the answer of part A:

`\begin{gathered} p=5 \\ q=3 \\ r=2 \end{gathered}`

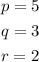
b) if the graph is reflected about to y-axis, write the equation of the curve​

So, the equation of the function will be:

`f(x)=-(3+5(x-2))`

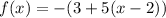
simplifying the equation

So,

`f(x)=-3-5(x-2)`