Question

Calculate the difference quotients and approximate the slope of the tangent line

Answer 1

Remember that

the difference quotient is equal to

`(H(x+h)-H(x))/(h)`

we have

H(x)=(1/3)x^2-2

so

`((1)/(3)(x+h)^2-2-\lbrack(1)/(3)x^2-2\rbrack)/(h)`
`((1)/(3)(x^2+2xh+h^2)-2-(1)/(3)x^2+2)/(h)`

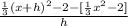
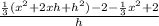
Simplify

`((1)/(3)x^2+(2)/(3)xh+(1)/(3)h^2-2-(1)/(3)x^2+2)/(h)`
`(+(2)/(3)xh+(1)/(3)h^2)/(h)=(2)/(3)x+(1)/(3)h`

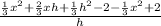
For h=0.1

`(2)/(3)x+(1)/(3)(0.1)=(2)/(3)x+(1)/(30)`

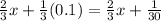
For h=0.01

`(2)/(3)x+(1)/(3)(0.01)=(2)/(3)x+(1)/(300)`

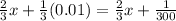
For h=0.001

`(2)/(3)x+(1)/(3)(0.001)=(2)/(3)x+(1)/(3,000)`

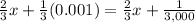
Find out the slope at the point (6,10)

For x=6

`(2)/(3)(6)+(1)/(3)h=4+(1)/(3)h`

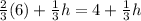
For h=0.1

slope is

4+1/30=4+0.033333=4.033333

For h=0.01

4+1/300=4+0.003333=4.003333

For h=0.001

4+1/3,000=4+0.000333=4.000333

the slope is 4 at point (6,10)