(a)
The given equation of a line is,
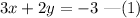
The general equation of a straight line is given by,
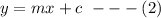
Here, m is the slope of the line and c is the y intercept.
Rewrite equation (1) into the form of equation (2).
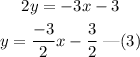
Comparing equations (1) and (3), we get the slope of the line m=-3/2.
Two parallel lines has the same slope. So, the slope of a line parallel to the line 3x+2y=-3 with slope m=-3/2 is -3/2.
(b)
The slope of a line perpendicular to the line with slope m=-3/2 is,
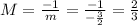
Therefore, the slope of a line perpendicular to 3x+2y=-3 with slope m=-3/2 is 2/3.