Question

Do I have to do Greatest Common denominator or do I factor it, or do I have to do AC grouping bc A doesn’t equal 1

Answer 1

The quadratic expression is

`9y^2+12y-5`

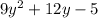
Step 1: We will have to get two numbers that we will multiply to get (-45)

`9*-5=-45`

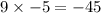
And the same two numbers must be added together to get

`+12`

The numbers are ( -3 and +15)

`\begin{gathered} -3*15=-45 \\ -3+15=+12 \end{gathered}`

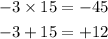
To get the two factors, we will need to list out all the factors of -45

Factors of -45 are

`\begin{gathered} 1,3,5,9,15,45 \\ -1,-3,-5,-9,-15,-45 \end{gathered}`

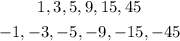
Note: list the negative of each factor. This will allow us to find all possible combinations.

The factors below will multiply to give a product of -45

`\begin{gathered} 1.(-45)=-45 \\ 3.(-15)=-45 \\ 5.(-9)=-45 \\ (-1).45=-45 \\ (-3).15=-45 \\ (-5).9=-45 \end{gathered}`

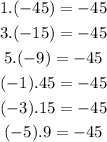
Now lets add up each pair and see which will give us +12

`\begin{gathered} 1+(-45)=-44 \\ 3+(-15)=-12 \\ 5+(-9)=-4 \\ -1+45=+44 \\ -3+15=+12 \\ -5+9=+4 \end{gathered}`

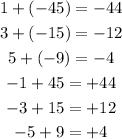
From the table, we can see that the two numbers -3 and 15 add to 12 (the middle coefficient).

So the two numbers -3 and 15 both multiply to -45 and add to 12

Now replace the middle term 12x with -3y + 15y. Remember, -3 and 15 add to 12. So this shows us that -3y + 15y=12y

`\begin{gathered} 9y^2+12y-5 \\ =9y^2-3y+15y-5 \end{gathered}`

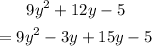
Group the terms into two pairs

`\begin{gathered} (9y^2-3y)+(15y-5) \\ \end{gathered}`

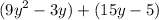
Factor out the G.C.F 3y from the first group and the G.C.F 5 from the second group

`\begin{gathered} (9y^2-3y)+(15y-5) \\ 3y(3y-1)+5(3y-1) \end{gathered}`

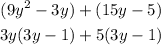
The goal of this step is to make the terms in the second parenthesis equal to the terms in the first parenthesis.

Combine like terms. Or factor out the common term (3x-1)

`\begin{gathered} 3y(3y-1)+5(3y-1) \\ (3y-1)(3y+5) \end{gathered}`

Hence,

9y² +12y -5 = (3y-1) (3y+5)