Question

Consider a triangle ABC like the one below. Suppose that A = 31°, C = 52°, and b = 29. (The figure is not drawn to scale.) Solve the triangle.Round your answers to the nearest tenth.If there is more than one solution, use the button labeled "or".

Answer 1

a = 15, b = 29 and c = 23

A= 31º B= 97º C= 52º

1) To solve a triangle is to find out its angles and measures. Since we have angle A= 31º, C =52º, and b= 29 we can solve this by using the Sum of Interior angles and the Law of sines:

`(a)/(\sin(A))=(b)/(\sin(B))=(c)/(\sin(C))`

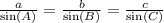
2) The sum of the interior angles of a Triangle is 180º So, we can find angle B this way

∠A +∠B+∠C= 180º

31º +∠B +52º = 180º

∠B + 83º = 180º Subtract 83 from both sides

∠B =97º

`\begin{gathered} (29)/(\sin(97))=(c)/(\sin(52)) \\ \\ c=(29\cdot\sin(52))/(\sin(97))\Rightarrow c\approx23 \end{gathered}`

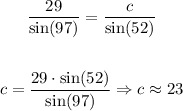
2.2) Now we can find the leg "a "

`\begin{gathered} (a)/(\sin (31))=(29)/(\sin (97)) \\ a\cdot\sin (97)\text{ = 29 }\cdot\sin (31) \\ a=(29\cdot\sin (31))/(\sin (97))\Rightarrow a\approx15 \end{gathered}`

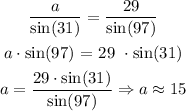
3) Hence, the answer is

a = 15, b = 29 and c = 23

A= 31º B= 97º C= 52º