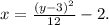Question

The directrix and focus of a parabola are shown on the graph. Which is an equation for the parabola?X=-5

Answer 1

From the graph, we notice that the parabola has to be a horizontal parabola with vertex at:

`(-2,3),`

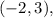
that opens to the right.

Recall that the standard form of a horizontal parabola is:

`\mleft(y-k\mright)^2=4p\mleft(x-h\mright)\text{.}`

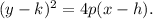
Where, (h,k) are the coordinates of the vertex, and p is the distance to the vertex to focus.

Substituting the vertex in the above equation, we get:

`(y-3)^2=4p(x-(-2))\text{.}`

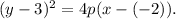
From the diagram, we get that:

`p=3.`

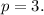
Therefore:

`(y-3)^2=12(x+2)\text{.}`

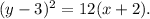
Solving the above equation for x, we get:

`\begin{gathered} ((y-3)^2)/(12)=x+2, \\ x=((y-3)^2)/(12)-2. \end{gathered}`

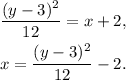
Answer:

`x=((y-3)^2)/(12)-2.`