Question

Can you please help me out with a question

Answer 1

To solve this problem we first need to calculate the total area of the circle, then calculate the area for the circular sector of 15 degrees and subtract the two areas.

The area of the circle is given by:

`A_{\text{circle}}=\pi\cdot r^2`

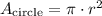
For this circle we have a radius of 9 m, therefore:

`A_{\text{circle}}=\pi\cdot(9)^2=81\cdot\pi\text{ square meters}`

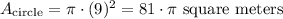
The area of a circular sector can be found by using the following expression:

`A_{\text{sector}}=(\theta\cdot\pi\cdot r^2)/(360)`

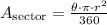
For this sector the angle is 15 degrees and the radius is 9 m, therefore:

`A_{\text{sector}}=(15\cdot\pi\cdot9^2)/(360)=3.375\pi\text{ square meters}`

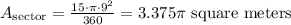
The area for the shaded sector is the subtraction between the two areas above. We have:

`A_{\text{shaded}}=81\pi-3.375\pi=77.625\pi\text{ =}243.866\text{ square meters}`

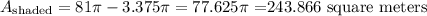
The correct option is B, the area of shaded sector is 243.866 square meters.