Question

Solving Real-World QuadraticsInstructions: For the following real-world problem, solve using anymethod. Use what you've learned to determine which methodwould be best. Put your answer in the context of the problem anddetermine the appropriate final answer.Jason is working on improving his golf game, specifically hischipping from the fringe. If his current arc of the ball and where itlands on the green can be modeled by the equation- 2x2 + 48x.= 0 where x is the distance in yards from Josh tothe ball.The two solutions to the equation are: x =orIn the context of this problem, which solution makes sense as thedistance from Jason where the ball lands? r =yardsCheck

Answer 1

-2x^2+48x = 0

The expression is written in the form:

ax^2 + bx + c = 0

Where:

a = -2

b= 48

c= 0

Apply the quadratic formula:

`\frac{-b\pm\sqrt[]{b^2-4\cdot a\cdot c}}{2\cdot a}`

Replacing;

`\frac{-48\pm\sqrt[]{48^2-4\cdot-2\cdot0}}{2\cdot-2}`
`\frac{-48\pm\sqrt[]{2304}}{-4}`
`(-48\pm48)/(-4)`

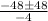
Positive;

`(-48+48)/(-4)=(0)/(-4)=0`

Negative:

`(-48-48)/(-4)=(-96)/(-4)=24`

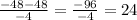
The 2 solutions are x =0 or x =24

In the context of the problem, 24 makes sense since 0 means no distance.