Question

A 1 kg block with initial velocity 4 m/s collides elastically with a stationary 2 kg block. After the collision, the 1 kg block has a velocity of -1.33 m/s. Determine the speed of the 2 kg block after the collision.

Answer 1

v₂ = 2,665 m / s

Step-by-step explanation:

This is an exercise of conservation of the momentum, we define a system formed by the two cars, so that the forces during the collision are internal and the moment is conserved.

Initial instant. Before the crash

p₀ = m v_o

Final moment. Right after the crash

p_f = m v₁ + M v₂

where the first car uses subscript 1 and has a mass m = 1 kg and the second car uses the subscript 2 with a mass M = 2kg

how the moment is preserved

p₀ = p_f

m v_o = m v₁ + M v₂

They indicate that the collision is elastic, therefore the kinetic energy is conserved, the velocity of the first car is v1 = -1.33 me / S

v₂ =
`( m ( v_o - v_1))/(M)`

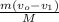
we calculate

v₂ =
`( 1 ( 4 + 1.33))/(2)`

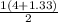
v₂ = 2,665 m / s