Question

3. A rectangle has an area of 24 square units. The width is 5 units less than the length. What is the length, in units, of the rectangle? a. 6 b. 8 c. 3 d. 19

Answer 1

b. 8

Step-by-step explanation:

Let the length of the rectangle = l units

The width is 5 units less than the length. Therefore:

• The width of the rectangle = (l-5) units

`\begin{gathered} \text{Area of a Rectangle}=\text{Length}* Width \\ \implies24=l(l-5) \end{gathered}`

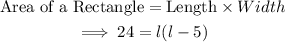
We solve the resulting equation for l.

`\begin{gathered} l^2-5l=24 \\ l^2-5l-24=0 \\ l^2-8l+3l-24=0 \\ l^{}(l-8)+3(l-8)=0 \\ (l+3)(l-8)=0 \\ l+3=0\text{ or }l-8=0 \\ l=-3\text{ or }l=8 \end{gathered}`

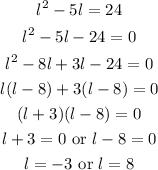
Since length cannot be negative, the length of the rectangle is 8 units.