Question

In the figure below, how many paper (in term of pi) is wasted if the largest possible circle with a diameter of d is cut out of the square?А. d - pi * d ^ 2B. d^ 2 pi/4C. (d ^ 2 * pi)/4 - d ^ 2D. (4d ^ 2 - d ^ 2 * pi)/4

Answer 1

Given:

Dimeter of the circle is d.

As circle lies inside the square. So, the length of the side of square is also d units.

The area of the circle is given as,

`\begin{gathered} A=\pi* r^2 \\ r=(d)/(2) \\ \Rightarrow\text{ Area of circle = }\pi*(d^2)/(4) \end{gathered}`

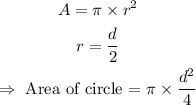
Now, the area of the square is,

`\begin{gathered} \text{Area of the square = side }^2 \\ \text{Area of the square = d}^2 \end{gathered}`

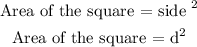
To find the area of paper that wasted when the circle is cut out of the square,

`\begin{gathered} A_p=\text{ Area of the square-Area of the circle} \\ =d^2-\pi*(d^2)/(4) \\ =d^2-(\pi(d^2))/(4) \\ =(4d^2-\pi(d^2))/(4) \end{gathered}`

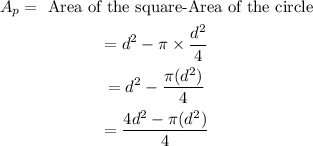
Answer: option D) is correct.