Question

Miriam has 40 meters of fencing to build a pen for her dog, One side of the pen does not need any fencing because a side of the garage will be used in place of one side of fencing. 1. What width will result in the greatest possible area of the dog pen? 2. What is the greatest possible area of the dog pen? 3. What length will result in the greatest possible area of the dog pen?

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Write the formula for finding the perimeter of the fence

`\begin{gathered} P=2L+2W \\ \text{where L is the length and W is the Width} \end{gathered}`

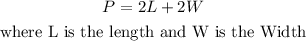
STEP 2: Write the dimensions of the Fence

`\begin{gathered} \text{The perimeter is 40m} \\ \text{Therefore,} \\ 2L+2W=40 \\ \text{ Making W the subject of the formula,} \\ 2W=40-2L \\ W=(40-2L)/(2) \\ W=20-L \\ \\ The\text{ length and the Width of the fence will be:} \\ L\text{ and 20-L} \end{gathered}`

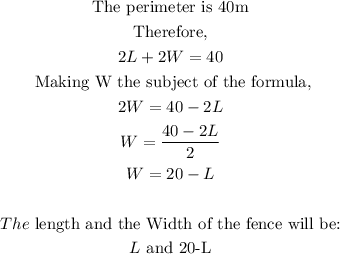
Hence, the width will result in the greatest possible area of the dog pen is:

`20-L`

STEP 3: Calculate the greatest possible area of the dog pen

`\begin{gathered} \text{Area}=\text{Length}* width \\ \text{Area}=L*(20-L) \\ \text{Area}=20L-L^2 \end{gathered}`

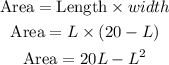
STEP 4: Calculate the length will result in the greatest possible area of the dog pen