Question

Write an equation for the quadratic that passes through:  (0,9),(−6,9), (−5,4)

Answer 1

The general form of a quadratic is:

`y=ax^2+bx+c`

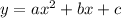
We need to plug in the 3 pair of points into "x" and "y" and simultaneously solve the 3 equations for a, b, and c.

Putting (0,9):

`\begin{gathered} 9=a(0)^2+b(0)+c \\ c=9 \end{gathered}`

Putting (-6,9):

`\begin{gathered} 9=a(-6)^2+b(-6)+c \\ 9=36a-6b+9 \\ 36a=6b \\ a=(6b)/(36) \\ a=(b)/(6) \end{gathered}`

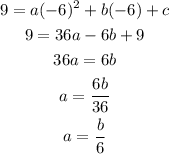
Putting (-5,4):

`\begin{gathered} 4=a(-5)^2+b(-5)+9 \\ 4=25a-5b+9 \\ 25a-5b=-5 \end{gathered}`

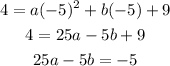
We substitute a=b/6 into this equation and solve for b:

`\begin{gathered} 25((b)/(6))-5b=-5 \\ (25b)/(6)-5b=-5 \\ (25b-30b)/(6)=-5 \\ -5b=-30 \\ b=6 \end{gathered}`

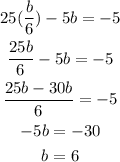
Thus, "a" will be:

`\begin{gathered} a=(b)/(6) \\ a=(6)/(6) \\ a=1 \end{gathered}`

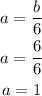
Thus, we have a = 1, b = 6, c = 9

The equation is:

`\begin{gathered} y=1x^2+6x+9 \\ y=x^2+6x+9 \end{gathered}`