Question

22. QRST is a rectangle. If RU = 3x - 6 and UT = x + 9, find x and the length of QS.RUx= 5QS =TS

Answer 1

We are given two lengths of the rectangle:

RU=3x-6

UT=x+9

These two lengths are shown in the following diagram:

Since this is a rectangle, the lengths of RU and UT must be equal:

`RU=UT`

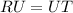
Thus

`3x-6=x+9`

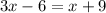
We need to solve this equation for x.

We start by subtracting x to both sides of the equation:

`\begin{gathered} 3x-x-6=9 \\ 2x-6=9 \end{gathered}`

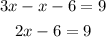
Now, add 6 to both sides:

`\begin{gathered} 2x=9+6 \\ 2x=15 \end{gathered}`

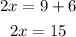
Finally, divide both sides by 2:

`\begin{gathered} (2x)/(2)=(15)/(2) \\ x=7.5 \end{gathered}`

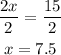
We have the value of x: x=7.5

Now we have to find the length of QS. Since QS and RT are diagonals of the same rectangle, they have to be equal:

`RT=QS`

This means that we can find RT by adding RU and UT, and the result will be equal to QS:

`QS=RU+TU`

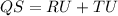
substituting the given expressions for RU and TU:

`QS=3x-6+x+9`

And now, substitute x=7.5 and solve for QS:

`QS=3(7.5)-6+7.5+9`
`\begin{gathered} QS=22.5-6+7.5+9 \\ QS=33 \end{gathered}`

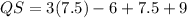
Answer:

x=7.5 and QS=33