To answer this question we will use the following property:

Subtracting 6 from the given inequality we get:
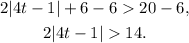
Dividing the above inequality by 2 we get:
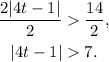
Then:
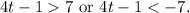
Solving the above inequalities we get:
1)
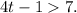
Adding 1 to the above inequality we get:

Dividing the above by 4 we get:
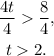
The above inequality in interval notation is:
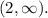
2)
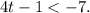
Adding 1 to the above inequality we get:
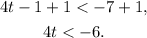
Dividing the above result by 4 we get:
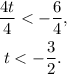
The above inequality in interval notation is:

Answer:
