Question

How does the multiplicity of a zero determine the behavior of the graph at that zero? the drop down options are: is tangent to, crosses straight through, and crosses though while hugging

Answer 1

The graph of the function crosses straight through the x-axis at -6.

The graph of the function is tangent to the x-axis at 0.

The graph of the function crosses straight through the x-axis at 1.

The graph of the function crosses through while hugging the x-axis at 4.

How to determine the true statements about the polynomial function

From the question, we have the following parameters that can be used in our computation:

Zeros of -6, 0 (multiplicity of 2), 1, and 4 (multiplicity of 3).

By definition:

• When the multiplicity is 1, the graph crosses through the x-axis
• When the multiplicity is 2, the graph is tangent to the x-axis
• When the multiplicity is 3, the graph crosses through while hugging the x-axis

Answer 2

Given: A seventh-degree polynomial function has zeros of -6, 0 (multiplicity of 2), 1, and 4 (multiplicity of 3).

Required: To determine the behavior of the graph at the zeros.

Explanation: The given seventh-degree polynomial can be represented as

`\left(x+6\right)\left(x-0\right)^2\left(x-1\right)(x-4)^3`

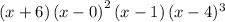
Now, the graph will cross straight through at x=-6 and x=1.

We have an odd multiplicity at x=4; hence the graph will cross through while hugging.

We have an even multiplicity at x=0; therefore, the graph will be tangent.

Here is the graph of the given function-

Final Answer: The graph will cross straight through at x=-6 and x=1,

the graph will cross through while hugging at x=4,

the graph will be tangent at x=0.