Question

For the given expression, which whole number (w) will make the expression true? 5 W 6 8

A. 3
B. 4
C. 5
D. 7

Answer 1

For the given expression, which whole number (w) will make the expression true is 4. Thus the correct option is B.

Step-by-step explanation:

To find the whole number (w) that makes the expression true
`- \(5^w * 6 = 8\)`, we can break it down step by step.

Starting with the expression
`\(5^w * 6 = 8\)`, we need to isolate the variable
`\(w\)`. First, divide both sides by 6 to isolate the term with the variable:

`\[ (5^w * 6)/(6) = (8)/(6) \]`

Simplifying, we get
`\(5^w = (4)/(3)\).`

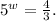
Now, take the logarithm (base 5) of both sides to solve for w:

`\[ \log_5(5^w) = \log_5\left((4)/(3)\right) \]`

Simplifying further, we get
`\(w = \log_5\left((4)/(3)\right)\).`

Now, evaluate the logarithm using common logarithm rules.

`\[ w = (\log((4)/(3)))/(\log(5)) \]`

By calculating, we find that w is approximately 0.585.

Since w needs to be a whole number, the closest whole number is 1. Therefore, the answer is
`\(w = 1\)`.