The given function is
f(x) = 6x^4 + 6
The formula for the limit is shown below
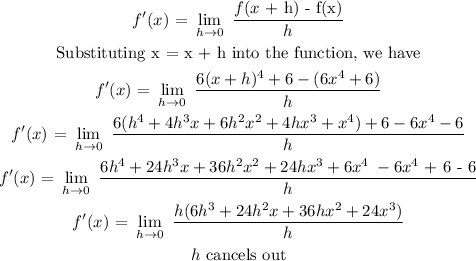
Evaluating the limit at h = 0, we would substitute h = 0 into 6h^3 + 24h^2x + 36hx^2 + 24x^3
It becomes
6(0)^3 + 24(0)^2x + 36(0)x^2 + 24x^3
The derivative is 24x^3
f'(x) = 24x^3
This is the slope of the tangent line is at x = 2
By substituting x = 2 into f'(x) = 24x^3, it becomes
f'(2) = 24(2)^3 = 192
To find the y coordinate of the point, we would substitute x = 2 into
f(x) = 6x^4 + 6
y = 6(2)^4 + 6 = 102
Thus, the x and y coordinates are (2, 102) and the slope is 192
The equation of the line in the point slope form is
y - y1 = m(x - x1)
Thus, the equation of the tangent is
y - 102 = 192(x - 2)