Question

The two shorter sides of a right triangle measure 18 ft and 24 ft. What is the measure in feet of the third side?

Answer 1

We have that in a right triangle, the larger side is the hypothenuse since the sum of the others angles must be equal to 90. Thus, we can apply the Pythagorean Theorem to solve this question.

The legs of the triangle are a = 18 ft, b = 24 ft, and c = ?.

Then, applying the Pythagorean Theorem, we have (without using units):

`c^2=a^2+b^2\Rightarrow c^2=(18)^2+(24)^2\Rightarrow c^2=324+576\Rightarrow c^2=900`

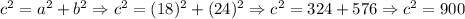
Then, taking the square root to both sides of the equation, we have:

`\sqrt[]{c^2}=\sqrt[]{900}\Rightarrow c=30`

Then, the measure of the third side (hypothenuse) is c = 30 ft.