Question

Determine the domain and range of the quadratic function. f(x)=−2(x+8)^2−4

Answer 1

`\begin{gathered} \text{Given} \\ f(x)=-2(x+8)^2-4 \end{gathered}`

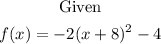
Since the given is a polynomial with a degree of 2, there are no restrictions to its domain. The domain therefore is

`\text{Domain: }(-\infty,\infty)`

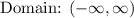
The given function is in the vertex form

`\begin{gathered} f(x)=a(x-h)^2+k \\ \text{where} \\ (h,k)\text{ is the vertex} \end{gathered}`

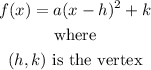
By inspection, we determine that the vertex of the function is at (-8,-4), and since a = -2, then the parabola opens up downwards. This implied that its output peaks at y = -4, and the graph continues towards negative infinity.

We can conclude therefore that the range is

`\text{Range: }(-\infty,-4\rbrack`