Question

A pyramid has a square base of 120ft. On a side. The four slant faces are all congruent isosceles triangles with base angles 55 degrees. Find the height of the pyramid?

Answer 1

Our first approach will be to get the slant height, i.e. the sides of the triangle. We can get this via the sine rule as:

We can get the side of the isosceles to be:

`\begin{gathered} (120)/(\sin70)=(x)/(\sin 55) \\ x=(120\sin 55)/(\sin 70)=104.6ft \end{gathered}`

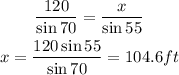
Now we need to find the perpendicular height.

To do that, we need to find the length of the diagonal of the base. We will apply the Pythagoras theorem.

This is given as:

`\begin{gathered} d=\sqrt[]{o^2+a^2} \\ \text{ Where:} \\ o=\text{opposite = length of base} \\ a=\text{adjacent = length of base} \end{gathered}`
`\begin{gathered} d=\sqrt[]{120^2+120^2} \\ d=170\text{ ft} \end{gathered}`

Next, we plot the triangle that will help us get our perpendicular height.

We now find h.

`\begin{gathered} h=\sqrt[]{104.6^2-85^2} \\ h=60.96\text{ ft} \end{gathered}`

The perpendicular height is 60.96 ft