Question

QUESTION 16Find anequation of the circle that satisfies the given conditions.Radius 6 and center (3.-5)

Answer 1

A circle can be represented by the following equation:

`\mleft(x-h\mright)^2+(y-k)^2=r^2`

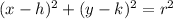
Where the radius is r and the center is (h, k).

Using the radius 6 and the center (3, -5), we have that:

`\begin{gathered} (x-3)^2+(y-(-5))^2=6^2 \\ (x-3)^2+(y+5)^2=36 \end{gathered}`

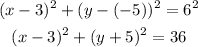
So the equation of the circle that satisfies radius = 6 and center = (3, -5) is:

(x-3)^2 + (y+5)^2 = 36