Given data:
The first set of equations are x+y=4, and x=6.
The second set of equations are 3x-y=12 and y=-6.
The point of intersection of first set of te equations is,
6+y=4
y=-2
The first point is (6, -2).
The point of intersection of second set of te equations is,
3x-(-6)=12
3x+6=12
3x=6
x=2
The second point is (2, -6).
The equation of the line passing through (6, -2) and (2, -6) is,
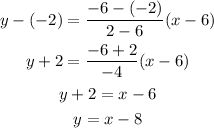
Thus, the required equation of the line is y=x-8.