Question

What is the quotient of this expression?

(x^4 - 3x + 2x^2 - 5x + 1)/
x^2 – 5
A) x^2 - 3
B) x^2 - 3x + 1
C) x^2 - 3x - 1
D) x^2 - 3x + 3
E) x^2 - 3x - 3

Answer 1

C)
`x^2 - 3x - 1` because The quotient of the given expression is
`\(x^2 - 3x - 1\)`because polynomial long division results in this quotient after dividing
`\(x^4 - 3x + 2x^2 - 5x + 1\) by \(x^2 - 5\).`

Step-by-step explanation:

To find the quotient of the given expression, we can perform polynomial long division. Divide the leading term of the numerator,
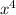`x^4`, by the leading term of the denominator,
`x^2`, which gives
`x^2.`Multiply the entire denominator,
`x^2 - 5, by x^2`, and subtract this result from the numerator. Continue this process to obtain the quotient
`x^2 - 3x - 1.`

Polynomial long division involves dividing each term of the numerator by the first term of the denominator. In this case, the leading term of the numerator, x^4, divided by the leading term of the denominator, x^2, gives x^2. Multiply the entire denominator by x^2, subtract this from the numerator, and repeat the process.

First step:
`\( (x^4 - 3x + 2x^2 - 5x + 1)/(x^2 - 5) \)`

Divide
`\(x^4\) by \(x^2\), which gives \(x^2\).`

Multiply the entire denominator by
`\(x^2\): \(x^2(x^2 - 5) = x^4 - 5x^2\).Subtract this from the numerator: \(x^4 - 3x + 2x^2 - 5x + 1 - (x^4 - 5x^2) = 2x^2 - 3x + 5x^2 + 1\).`

Repeat the process with the new expression:
`\( (2x^2 - 3x + 1)/(x^2 - 5) \).Divide \(2x^2\) by \(x^2\), which gives \(2\).Multiply the entire denominator by \(2\): \(2(x^2 - 5) = 2x^2 - 10\).`

Subtract this from the numerator:
`\(2x^2 - 3x + 1 - (2x^2 - 10) = -3x + 11\).Now, \( (-3x + 11)/(x^2 - 5) \).This process continues until we get the quotient \(x^2 - 3x - 1\).`