Question

Three years ago Maya was eleven times as old as her daughter Fiona. In four years time Maya will be four times as old as Fiona. How old are they now?

Answer 1

Let's call M the present age of Maya, and F the present age of Fiona.

Three years ago Maya was eleven times as old as Fiona. At that time, Maya's age was M-3, and Fiona's age was F-3. Thus, we have:

`M-3=11(F-3)`

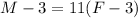
Also, in four years Maya will be four times as old as Fiona. At that time, Maya's age will be M+4, and Fiona's age will be F+4. Thus, we have:

`M+4=4(F+4)`

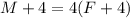
Now, we need to solve the system of those two equations to find M and F. Subtracting the second equation from the first, we obtain:

`\begin{gathered} M-3-(M+4)=11F-33-(4F+16) \\ \\ M-3-M-4=(11-4)F-33-16 \\ \\ -7=7F-49 \\ \\ -7+49=7F-49+49 \\ \\ 42=7F \\ \\ (42)/(7)=(7F)/(7) \\ \\ 6=F \\ \\ F=6 \end{gathered}`

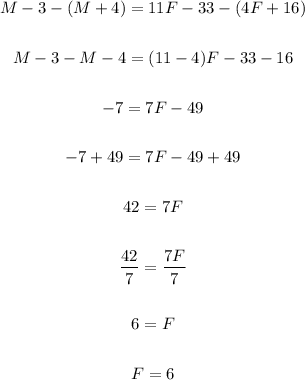
Now, we can use the above result to find M:

`\begin{gathered} M+4=4(6+4) \\ \\ M+4=40 \\ \\ M+4-4=40-4 \\ \\ M=36 \end{gathered}`

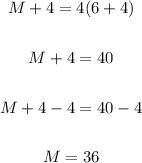
Therefore, now Maya is 40 years old and Fiona is 6 years old.