Question

A man can jump 12m on the surface of the moon. At what height can he jump on the surface of the earth?

Option 1: 3.6m
Option 2: 1.2m
Option 3: 36m
Option 4: 120m

Answer 1

The man can jump approximately 1.2m on the surface of the Earth.

Step-by-step explanation:

On the moon, the gravitational acceleration is about 1/6th of that on Earth. The height reached in a jump is determined by the formula
`\( h = (1)/(2)gt^2 \)`, where
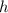`\( h \)` is the height,
`\( g \)` is the acceleration due to gravity, and
`\( t \)` is the time of flight. As the gravitational acceleration on the moon is 1/6th of that on Earth, the time of flight
`(\( t \))` will remain nearly the same for the same jump.

Let
`\( h_{\text{moon}} \)` be the height of the jump on the moon (12m) and
`\( g_{\text{moon}} \)` be the gravitational acceleration on the moon. We can set up the following equation:

`\[ h_{\text{moon}} = (1)/(2)g_{\text{moon}}t^2 \]`

Similarly, let
`\( h_{\text{earth}} \)` be the height of the jump on Earth and
`\( g_{\text{earth}} \)` be the gravitational acceleration on Earth. The equation for the jump on Earth is:

`\[ h_{\text{earth}} = (1)/(2)g_{\text{earth}}t^2 \]`

Dividing the two equations, we get:

`\[ \frac{h_{\text{earth}}}{h_{\text{moon}}} = \frac{g_{\text{earth}}}{g_{\text{moon}}} \]`

Substituting the values, we find:

`\[ \frac{h_{\text{earth}}}{12} = (9.8)/(1.625) \]`

Solving for
`\( h_{\text{earth}} \)`, we get
`\( h_{\text{earth}} \approx 1.2 \)` meters.