Question

Find the missing angletan x= 0.9004

Answer 1

Step-by-step explanation

We are told that the tangent of an angle x is equal to 0.9004. In order to solve this equation for x we can use the arctangent function that has this property:

`\tan^(-1)(\tan x)=x`

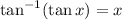
Then we can apply the arctangent to both sides of our equation:

`\begin{gathered} \tan^(-1)(\tan x)=\tan^(-1)0.9004 \\ x=\tan^(-1)0.9004 \end{gathered}`

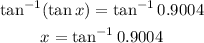
So our angle is the arctangent of 0.9004 which can be found using a calculator. However calculators oftenly work with radians and we need to express x in degrees. In order to transform the result from radians to degrees we have to perform the following operation:

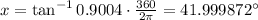
`x=\tan^(-1)0.9004\cdot(360)/(2\pi)=41.999872^(\circ)`Answer

Wheter we round to the nearesth tenth, hundreth or thousanth the answer is 42°.