Question

The base of the pyramid is a square.158Perimeter of the base =Area of the base =Slant height =Lateral area =square unitsSurface area =square unitsBlank 1:Blank 2:Blank 3:Blank 4:Blank 5:

Answer 1

The pyramid has a square base.

i) Perimeter of the base implies the perimeter of the square.

Perimeter of a square is given as:

`\begin{gathered} P=4L \\ L=8 \\ P=4*8 \\ =32 \end{gathered}`

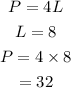
ii) Area of the base implies the area of the square.

Area of a square is given as:

`\begin{gathered} A=L^2 \\ L=8 \\ A=8^2 \\ A=64 \end{gathered}`

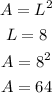
iii) The slant height can be obtained by using the pythagoras theorem.

From the diagram, the hypotenuse side is the unknown slant height, the other two(2) sides are of length 15 and 8.

Thus, we have:

`\begin{gathered} H^2=O^2+A^2\text{ (Pythagoras theorem)} \\ H^2=15^2+8^2 \\ H^2=225+64 \\ H^2=289 \\ H=\sqrt[]{289} \\ H=17 \end{gathered}`

Hence, the slant height is 17

iv) The lateral area of a square pyramid is the sum of the areas of all its 4 triangular side faces.

The area of a triangle is given as:

`\begin{gathered} A=(1)/(2)* Base* Height \\ \text{Base}=8;\text{ Height=15} \\ A=(1)/(2)*8*15 \\ A=(120)/(2) \\ A=60 \\ \text{Hence, the lateral area is 4}*60\text{ ( since there are 4 triangular faces)} \\ \text{Lateral area= 240} \end{gathered}`

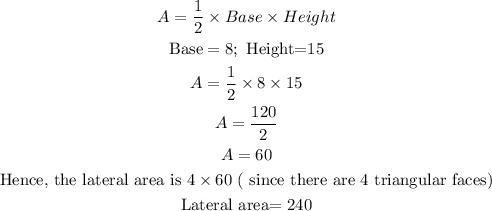
v) The surface area is the sum of the lateral area and the base area.

The lateral area has been obtained to be 240.

The base area has been obtained to be 64.

Thus, the surface area = 240 + 64

Hence, the surface area is 304