Question

Can you please help me answer this question thank you if it’s A, B C or D

Answer 1

Concept

In probability theory, the central limit theorem establishes that, in many situations, when independent random variables are summed up, their properly normalized sum tends toward a normal distribution even if the original variables themselves are not normally distributed.

Given:

period of record = 5 years

mean daily revenue = $5400

Standard deviation = $54

We want to identify which of the options perfectly describes the sampling distribution of the sample mean supposing that 36 days are randomly selected.

Using the central limit theorem, we know that regardless of the distribution one samples from if the population mean and standard deviation are:

`\begin{gathered} population\text{ mean (}\mu) \\ \text{Standard devaition (}\sigma) \end{gathered}`

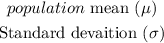
then, the mean is approximately normally distributed and has a value equal to the population mean, while the standard deviation of the sample means is:

`\frac{\sigma}{\sqrt[]{n}}`

Hence the standard deviation of the sample means is:

`\begin{gathered} =\text{ }\frac{54}{\sqrt[]{36}} \\ =\text{ \$9} \end{gathered}`

We can conclude that the distribution is normal with a mean of $5400 and a standard deviation of $9

Answer: Option B