Question

For students who fint enroled in two-year public instzutions in a recent semester, the proportion who eamed a bachelors degree, within six years was 0.386. The president of a certain junior celtoge beloves that the proportion of students who enrol in her instation have a lower completion rate. (a) State the null and altarnative hypothoses in words. (b) State the null and alternative hypotheses symbolicaly. (c) Explain what it would mean to make a Type I error. (d) Explain what it would mean to make a Type II error. (a) State the null typothesis in words. Choose the correct answer below. A. Arnong students who first enroll in two-year public institutions, the completion rate is 0.386. B. Among students who enrol at the certain junicr college, the completion rate is less than 0.366. C. Among students who eneoll at the certain puilor colege, the corrpletion rase is greater than 0.386. D. Among studants who enmoll at the certain junior colloge, the completion rate is 0.386. State the ahernative hypothesis in words. Choose the correct answer below. A. Among studenta who first aneoll in two-year public institutions, the comploton rate is 0.366. B. Among students who onroll at the certain jurior codlege. the compleich rate is loss than 0.356. C. Among students who enrol at the certain junior colege, the complotion rote is 0.386. D. Anong atudents who errol at the certain junice colege, the completion rase a greater nan 0.386. (b) 5 tate the hypotheses symbolically? (Type integors or docimais. Do not round. D. Among students who enroll at the certain jurior college, the completion rate is 0.396. State the alternative hypothesis in words. Choose the correct answer below. A. Among students who first enrot in two-year public institutions, the complotion rate is 0.386. B. Among students who enroil at the certain junior collego, the completion rale is less than 0.386. C. Ameng otudents who enroi at the certain juniot coliege, the complotion rate is 0.386. D. Among students who enroll at the cortain funior college, the completion rato is greater than 0.386. (b) State the hypotheses symbolicaly. (Type integere of decimals. Do not round.) (c) Vhat would it mean to make a Type 1 erroe? The president the bypothesis that the proportion of students who eurn a bachelor's degreo within six years is (Type invegers or dedimals. Do not round.) (d) What would it mean to make e Type Il erroe? The presidon the hypothesis that the propertion of students who eam a tachelors degroe within six years is when, in fact, the proportion is (Type intagars or decimass Do not round).

Answer 1

(a) Null Hypothesis: The completion rate among students at the certain junior college is 0.386; Alternative Hypothesis: The completion rate is less than 0.386.

(b) Symbolic Hypotheses:
`\(H_0: p = 0.386\),`
`\(H_1: p < 0.386\).`

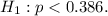
(c) Making a Type I Error: Incorrectly concluding a completion rate less than 0.386 at the certain junior college when it is 0.386 or higher.

(d) Making a Type II Error: Failing to detect a completion rate less than 0.386 at the certain junior college when it actually exists.

Step-by-step explanation:

(a) The null hypothesis
`(\(H_0\))` states that the completion rate for students at the certain junior college is 0.386, while the alternative hypothesis (
`\(H_1\))` asserts that the completion rate is less than 0.386. This sets up a one-sided test to determine if the president's belief about a lower completion rate is supported.

(b) Symbolically, the hypotheses are represented as
`\(H_0: p = 0.386\)`and
`\(H_1: p < 0.386\)`, where
`\(p\)`is the population proportion of students who earn a bachelor's degree within six years.

(c) Making a Type I Error involves rejecting the null hypothesis when it is true. In this context, it would mean concluding that the completion rate at the certain junior college is less than 0.386 when, in fact, it is 0.386 or higher.

(d) Making a Type II Error entails failing to reject the null hypothesis when the alternative is true. In this case, it would mean not detecting a lower completion rate at the certain junior college when, in reality, it is less than 0.386. Both types of errors have implications for the accuracy of the hypothesis test and decision-making.