Question

1. The diagram below, not drawn to scale, shows a flexible piece of paper in the shape of a sector of a circle with centre 0 and radius 15 cm. 22 Use . B А 126 0 15 cm C (a) Show that the perimeter of the paper is 63 cm. [3] (b) Calculate the area of the paper OABC. 121 (c) The paper is bent and the edges OA and OC are taped together so that the paper forms the curved surface of a cone with a circular base, ABC. (1) Draw a diagram of the cone formed, showing clearly the measurement 15 cm, the perpendicular height, h, and the radius, r, of the base of the cone. [1] (ii) Calculate the radius of the circular base of the cone. 121 (iii) Using Pythagoras' Theorem, or otherwise, determine the perpendicular height of the resulting cone. 121

Answer 1

Given

Circle of radius 15 cm and angle at the centre equal to 126 degree.

Find

(a) Perimeter of the paper is 63cm.

(b) Area of the paper OABC

(c) i) Draw a cone

ii) radius of circular base

iii) determine the height

Step-by-step explanation

(a)

Perimeter of sector = Arc length ABC + AO + OC

Arc Length of ABC =

`\begin{gathered} (\theta)/(360)*2\Pi r \\ (126)/(360)*2*(22)/(7)*15 \\ 33 \end{gathered}`

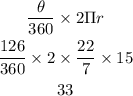
so , perimeter = 33 +15 +15 = 63

Hence we proved that perimeter is 63 cm

(b) Area of sector =

`\begin{gathered} (\theta)/(360)*\Pi r^2 \\ (126)/(360)*(22)/(7)*15*15 \\ 247.5 \end{gathered}`

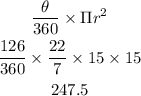
(c) i)

ii) Circumference of base =

`\begin{gathered} 2\Pi r=\text{33} \\ r=(33*7)/(2*22) \\ r=(21)/(4) \end{gathered}`

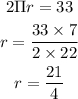
iii) l = 15 cm, r= 21/7

By pythagoras theorem,

`\begin{gathered} h^2=l^2-r^2 \\ h^2=15^2-((21)/(4))^2 \\ h=\text{ 14.05} \end{gathered}`

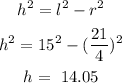
Final Answer

(a) 63

(b) 247.5