Final answer:
The solution of the inequality
![(\pi /2-e/3) ^((ln(-2cosx))\geq 11[0;2\pi]](https://img.qammunity.org/2024/formulas/mathematics/high-school/fhnammyctxscwuvnbwass9tpb47cym4nj8.png) is x ∈ (π/2, 3π/2)
is x ∈ (π/2, 3π/2)
None of the given options is correct
Step-by-step explanation:
The given inequality is:
![(\pi /2-e/3) ^((ln(-2cosx))\geq 11[0;2\pi]](https://img.qammunity.org/2024/formulas/mathematics/high-school/fhnammyctxscwuvnbwass9tpb47cym4nj8.png)
To solve this inequality, we need to follow these steps:
Step 1: Take the natural logarithm on both sides of the inequality.
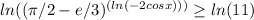
Step 2: Use the logarithm properties to simplify the left side of the inequality.
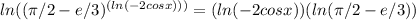
Step 3: Now, the inequality becomes:
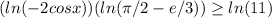
Step 4: We need to solve each part of the inequality separately.
For the first part, ln(-2cosx), the natural logarithm of a negative number is undefined. Therefore, we need to find the values of x for which -2cosx > 0.
Since the cosine function is positive in the first and second quadrants, we have:
0 < x < π/2 and π < x < 3π/2
For the second part, ln(π/2 - e/3), we need to find the values of x for which π/2 - e/3 > 0.
Solving the inequality, we get:
π/2 > e/3
3π/2 > e
So, the values of x that satisfy
 are:
are:
π/2 < x < 3π/2
Step 5: Now, let's find the values of x that satisfy both parts of the inequality.
Combining the results from step 4, we have:
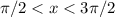
Therefore, the correct answer is: x ∈ (π/2, 3π/2)
So, none of the given options is correct
Your question is incomplete, but most probably the full question was:
![(\pi /2-e/3) ^((ln(-2cosx))\geq 11[0;2\pi]](https://img.qammunity.org/2024/formulas/mathematics/high-school/fhnammyctxscwuvnbwass9tpb47cym4nj8.png)
Please solve this
a)
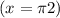
b)

c)
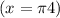
d)
